Chapter
13 Electric Field
1.
Give the definition of the electric field
2. Produce the equation stating Coulomb's Law
3. Find the electric field vecotr at a location due to one or more
point charges
4. Sketch the electric field due to a point charge
5. Calculate the force vector on a charge due to the electric field
at the charge's location
6. Calculate the field vector at a location given the force on a known
charge
7. Calculate the force vector between two charges
8. State the superposition principle
9. Sketch the electric field around a dipole
10. Approximate the electric field near a dipole, especially along
the axis, perpendicular to the axis, and far from the dipole
11. Calculate the force on a point charge due to a dipole
12. Calculate a dipole moment vector
13. Describe the forces on a dipole in a uniform electric field
Chapter
14 Matter and Electric Fields
1.
Apply the conservation of charge concept to a physical situation or
calculation
2. Sketch the distribution of charge inside and on the surface of
a neutral or charged conductor or insulator due to external electric
fields produced by other charges
3. Find the net electric field inside a conductor
4. Find the electric field inside a conductor solely due to the polarized
charges on its surface.
5. Formulate a method to determine if an object is charged or polarized.
Illustrate the differences between a charged and polarized solid sphere.
6. Determine the electrical forces between charged and neutral matter
7. Distinguish between conductors and insulators and the electrical
properties of each
8. Charge objects by contact or induction
9. Sparks in air will be discussed later in the semester
Chapter
15 Electric Field of Distributed Charges
1. Sketch and calculate the electric field due to various charge distributions,
eg. uniformly distributed charge on rod, ring, disk, capacitor, spherical
shell, solid sphere. (You need to be able to do this from a formula
you derive as well as from formulas that are provided.)
2. Sketch and calculate the electric field due to the superposition
of fields from various charge distributions, dipoles, and point charges.
3. Calculate the fringe field of a capacitor at some location.
4. Use VPython to numerically evaluate and display the electric field
due to a charge distribution.
5. Evaluate the net electric field at any point inside a hollow conductor.
6. Specify when to use exact and approximate formulas to calculate
the electric field.
7. Show how to approximate an exact formula for electric field under
specified conditions (observation location far away or very close,
etc.).
8. Give examples of charge configurations where the electric field
depends on distance as 1/r, 1/r^2, and 1/r^3.
Chapter
16 Electric Potential
1. Recall details of energy and potential energy learned in Mechanics
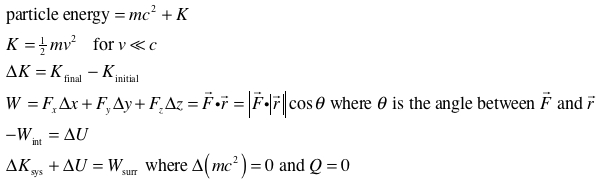
2. Calculate work done by an electric force on a moving particle
3. Determine the electric potential difference between two locations
in a uniform electric field, regardless of path
4. Find the change in electric potential energy of a charge which
sees a change in kinetic energy (and vice versa), regardless of path
5. Determine the sign of potential difference for a given situation,
without resorting to calculations
6. Determine the electric potential difference in a nonuniform electric
field
7, Add the potential differences across several regions with different
fields
8. Apply the general definition of potential difference to various
charge configurations
9. Recognize that potential difference is independent of path and
that the potential difference around a closed path is zero
10. Calculate the electric potential at a single location in three
dimensional space
11. Determine the potential difference between two points in a conductor
at equilibrium (zero) as well as between two points in or near an
insulator.
12. Determine the dielectric constant for an insulator
13. Find the reduction in potential difference because of the presence
of an insulator with a given dielectric constant
Chapter
17 Magnetic Field
1. Articulate the definition of electron current in a conductor
2. Use a compass to detect the magnetic field at various locations
near a current carrying wire
3. At various locations near a current-carrying wire, calculate the
magnitude of the magentic field by using a compass and trigonometry
(by comparison with the Earth’s horizontal field component of
~20 µT)
4. Calculate the cross product of two vectors, given either their
components or their magnitudes and the angle between them
5. Construct the magnetic field produced by moving charges using the
right hand rule. (Note that there are several versions of the RHR.
You should be familiar with each of them.)
6. Use the Biot-Savart law to find the magnitude and direction of
the magnetic field due to a moving charge
7. Articulate the difference between electron current and conventional
current
8. Derive and apply a formula to find the current in a conductor,
knowing its cross-sectional area, charge carrier density, and average
drift speed of the charges moving through it. Note that this could
be either an electron current or a conventional current.
9. Apply the Biot-Savart law for current-carrying wires of various
simple shapes like a short, straight piece of wire or a circular loop.
10. Sketch the magnetic field vectors at different locations near
the above current-carrying wires.
11. Find the magnitude and direction of the magnetic field on the
axis of a loop
12. Articulate when it is appropriate to use an approximation for
the above calculation
13. Calculate the magnetic dipole moment of a coil of wire
14. Compare the magnetic field generated by a current-carrying coil
of wire to that generated by a permanent magnet
15. Describe how the magnetic moments of a permanent magnet’s
orbiting atomic electrons generate the macroscopically observed field
16. Explain how orientation of magnetic domains impacts the observed
field around a magnet
Chapter 18 Microscopic View of Electric Circuits
1. Articulate the difference between “equilibrium” and “steady state” as the terms apply to metal wires used in circuits while describing: the location of excess charge, motion of mobile electrons, and the electric field insdie the conductor
2. Articulate the difference between emf and electrical potential difference
3. Compare the currents in different parts of a series circuit
4. Use charge and energy conservation (node and loop rules) to solve problems involving circuits
5. Use the Drude model to relate the drift speed of mobile charges and the electric field in a wire, given the wire’s charge carrier mobility
6. Compare the drift speeds of charge carriers in wires of different thicknesses
7. Sketch the surface charge distribution on the wires and resistors of a simple circuit and describe how the resulting electric field drives the electric current
8. Describe the transient and steady state charge distributions and electric fields present when a simple loop circuit is connected
9. Articulate how feedback maintains steady-state conditions in an electric circuit
10. Apply energy conservation (the loop rule) around a closed path in a circuit and sketch the potential changes around the circuit
11. Articulate the role of a battery in an electric circuit from a microscopic point of view
12. Use charge and energy conservation (node and loop rules) to solve problems involving circuits
Chapter 19 Capacitors, Resistors, and Batteries
1. Explain how a capacitor charges and stores charge
2. Describe the processes of charging and discharging a capacitor
3. Draw charge distributions and electric field in the wires of a circuit containing a capacitor charging or discharging at all times after connection
4. Relate the geometry of a parallel plate capacitor to the field inside the capacitor, the charge the capacitor can hold, and its capacitance
5. State and use the definition of capacitance
6. Relate current density and conductivity to find the electric field inside a wire
7. Articulate the difference between current and current density
8. Articulate the difference between resistance and resistivity
9. Derive I = | DV|/R from microscopic properties (without referring to notes)
10. Articulate the difference between “ohmic” and “non-ohmic” circuit elements. Explain how semiconductors fit into this categorization.
11. Solve series and parallel circuits for an unknown emf, current, or resistance
12. Calculate the power dissipated or provided by any circuit element
13. Graph potential (in volts) vs. location in the circuit
14. Explain how a real battery differs from an ideal battery
15. Connect ammeters and voltmeters correctly in a circuit
1. Identify which has more resistance and explain why that matters for how you connect it
2. Articulate and use the sign convention for ammeters and voltmeters
16. Calculate the capacitance of a capacitor through experimental observation
17. Sketch charge and current as a function of time for RC circuits
Chapter 20 Magnetic Force
1. Calculate the vector magnetic force on a moving charge
2. Describe the motion of a charged particle moving in a magnetic field
3. Relate the radius of curvature to the momentum of a particle in a magnetic field
4. Calculate the vector magnetic force on a short length of current-carrying wire
5. Use the full Lorentz Force to calculate the net force on moving charges
6. Explain, using equations, how a velocity selector works
7. Use the Hall Effect to determine the sign of the charge carriers for a given material
8. Draw the charge distribution on a conductor traveling in a magnetic field
9. Determine the direction and magnitude of the current in a motional-emf circuit
10. Calculate the power dissipated in a motional-emf circuit
11. Calculate the torque on a magnetic dipole
12. Calculate the potential energy for a magnetic dipole
Chapter 21 Patterns of Field in Space
1. Define flux, and articulate examples of flux that occur in everyday life.
2. Define electric flux, both in words and in a mathematical expression. Articulate the role of each of the following: direction of electric field with respect to the outward-going normal, the magnitude of the electric field, and the surface area.
3. State Gauss’s Law (for electricity) in discrete and continuous forms.
4. Explain the role of a Gaussian surface in evaluating Gauss’s Law.
5. Identify and explain specific situations where Gauss’s Law can be useful (and where it’s not helpful).
6. Use Gauss’s Law to find the electric field due to
a. A uniformly charged plate
b. A uniformly charged sphere
c. A point charge
d. A uniformly charged cylinder
7. Describe the dimensions of the Gaussian surface used to evaluate Gauss’s Law in Objective 6.
8. State Gauss’s Law for magnetism, and explain its implications.
9. State Ampere’s Law (for magnetism).
10. Explain why we shouldn’t use the phrase “magnetic flux” with Ampere’s Law
11. Explain what is meant by “Amperian path” and contrast it with a Gaussian surface.
12. Use Ampere’s Law to find the magnetic field due to
a. A thin wire
b. A thick wire
c. A solenoid
d. A toroid
13. Summarize each of Maxwell’s Equations in integral form by:
a. Stating the relationships between electric field, magnetic field, and charge density.
b. Explaining qualitatively what each equation means and its consequences.
c. Identify which of Maxwell’s Equations is known as the following: Ampere’s Law, Faraday’s Law, Gauss’s Law.
Chapter 22 Changing Magnetic Fields and Curly Electric Fields
1. Sketch the appearance (including direction at various locations) of the curly electric field due to a time-varying magnetic field.
2. Determine the direction of induced current in a conductor due to a non-Coulomb electric field.
3. Determine the magnetic flux through various areas.
4. Apply Faraday’s Law to find the emf due to a changing magnetic flux.
5. Calculate a motional emf in terms of the rate of change of magnetic flux.Explain the differences and similarities between the non-Coulomb electric field and the Coulomb electric field.
6. Apply the chain rule to evaluate how the magnetic flux changes with time.
Chapter 23 Electromagnetic Radiation
1. Explain the addition Maxwell made to Ampere’s Law and why it is needed.
2. Describe the various parts of each of Maxwell’s equations and how they are related.
3. Explain how an electromagnetic wave propagates through space, using Maxwell’s equations.
4. Describe the types of fields generated by a charge at rest, at constant velocity, and accelerating.
5. Calculate the energy density in electric and magnetic fields and electromagnetic radiation.
6. Determine the energy flux (the “Poynting vector) of an electromagnetic wave
7. Calcuate the momentum flux of an electromagnetic wave.
8. Describe how electromagnetic waves interact with matter
9. Explain all observations made of the “wireless” light bulb demonstration
10. Determine the direction and magnitude of the radiative electric and magnetic fields generated by an accelerated charge.